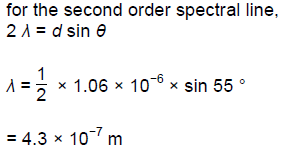Chapter C5 – AQA Physics A Level Year 1 Optics

C5.1 Refraction of light:

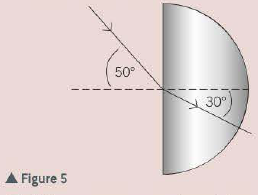
Page No. 69

Summary questions

1 (a)

i 20⁰

14.9°

ii 40⁰

28.9°

iii 60⁰.

40.6°

1 (b)

58.7°

2 (a)

(i) 30⁰

19.5°

(ii)60⁰

35.2°

2 (b)

i 35⁰

59.4°

ii 40⁰.

74.6°

1.53

34.5°

4 (a)

Sketch this arrangement and show that the angle of refraction of the light ray in the glass is 220.

4 (b)

i Show that the angle of incidence where the light ray leaves the glass prism is 380

72.6°

C5.2 More about refraction:

Page No. 72

Summary questions

1 (a)

0.040m

0.030m,

1 (b)

18.5°

2 (a)

1.97 x 10⁸ ms^-1

2.26 x 10⁸ ms^-1

2 (b)

3 (a)

25.4°

(b)

35°

4 (a)

Red 49.5°

Blue 50.8°

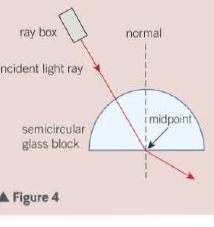
5.3 Total internal reflection:

Page No. 75

Summary questions

1 (a)

If the angle of incidence is greater than a certain limiting angle, called the critical angle.

When a ray of light in a medium of higher index of refraction approaches the other medium at an angle of incidence greater than the critical angle.

(b)i

41 °

49°

2 (a)

2(b)

34°

3 (a)

65°

3(b)

30°

45°

4(a)

A coherent fiber bundle is a collection of single fiber optic strands assembled together so that the relative orientation of the individual fibers is maintained throughout the length of the bundle.

The positions of ends of the fibres at the internal end need to map exactly to the same positions at the viewing end. In other words, the fibres cannot be allowed to “tangle up” as they go through the cable. Otherwise, the image would also be “tangled up”.

5.4 Double slit interference:

Page No. 78

Summary questions

1 (a)

The standard formula

W= (DX Wavelength)/s

W= Fringe seperation

D= distance of slit to the screen

s= distance between centre of the slits

Since D Represents the distance of the slit from the the screen, it is a proportional relationship to the fringe seperation(W). So, if the distance is decreased less spaced.

1(b)

If one slit is blocked, the pattern disappears. Even if the light is dim enough so that only single photons are travelling at any one time. Which is just weird – it seems that a photon can go through two slits at the same time.

2 (a)

550nm

3 (a)

0.9mm

4 (a)

0.75m

5.5 More about interference:

Page No. 81

Summary questions

1 (a)

b Describe the fringe pattern you would expect to observe in a.

2(a)

3 (a)

1.1 mm

5.6 Diffraction:

Page No. 84

Summary questions

1 (a)

The separation of the interference pattern fringes does not depend on the width of the slits.
Each slit produces its own diffraction pattern.
As the slit gets wider the diffraction pattern gets narrower. Where the two diffraction patterns overlap you get the interference pattern.
When the slits are narrow the diffraction spreads the light out and there is considerable overlap of the diffraction patterns and hence there are many fringes visible.
With wide slits and narrow diffraction patterns the light does not spread out as much and so the amount of overlap of the diffraction patterns is less so there are fewer fringes visible.

2(a)

6mm

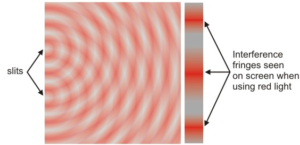
Red light interference pattern (λ = about 650nm)

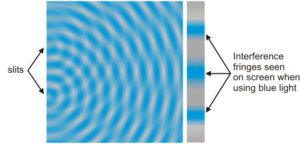
Blue light interference pattern (λ = about 450nm)

3 (a)

They are dimmer because they are contained within the envelope of the single slit pattern, and the single slit envelope intensity gets less at the outer edges.

(b)

The slits being made wider means that we are less certain as to the position of the photons as they traverse the slits, which basic quantum mechanics will dictate that uncertainty of momentum will increase. Because the momentum is more likely to be of higher value in these circumstances, the light has a greater inertia and so diffracts less. So there is a much smaller angle of diffraction in this case.

(c)

5.7 The diffraction grating:

Page No. 87

Summary questions

1(a)

10.9°, 22.2°

(b)

2(a)

(b)

0.58 (= 35′)

3 (a)

1090

(b)

69.9°

4 (a)

599mm^-1

4(b)

Practice questions:

Page No. 88-89

1 (a)

(i)

52 sin c = 1.35 sin 90 gives
Sin c = 0.888.
Hence c = 62.6 °

(ii)

light ray confined to core

angle of incidence > 62.6 °
more than one total internal reflection shown

b (i)

Total internal reflection takes place at the core-cladding boundary so the light rays stay in the core. If two fibres are in contact, light cannot pass from one fibre to the other because it is confined to the core of each fibre.
Without cladding, the cores would be in contact and light could pass from one fibre to another where they are in contact.
Light signals in each fibre would therefore not be secure.

b(ii)

Benefits; security camera intended to deter unauthorised access; data is more secure in transmission using optical fibre than other methods; image storage enables past images to be viewed if necessary

Drawbacks; security camera could be disconnected, stored images could be deleted or misused.

2(a)

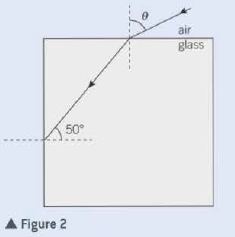
Ray drawn to show:

total internal reflection at right hand interior face of glass block
ray emerging from base of block into the air
refracted away from normal

2(b)

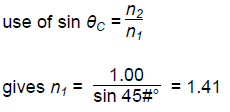
2(c)

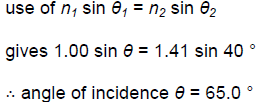
3(a )

(i)

ray refracting towards the normal on entry
Total internal reflection at the surface, several times along the fibre
Refraction away from the normal on emerging from the right hand end of the fibre

(ii)

the speed of light decreases when it enters the
glass fibre and increases again when it
emerges into the air

3(b)

(i)

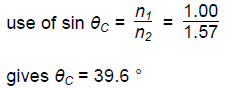
(ii)

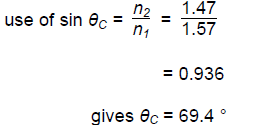
(iii)

Advantage of cladding:

the cladding protects the surface of the core
it also prevents cross-over between adjacent fibres

4 (a)

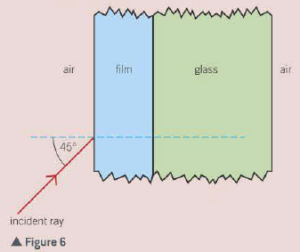
i. Coherent sources of light produce:

waves of the same wavelength (or frequency)
waves that have a constant phase difference (which may be zero)

4(b)(i)

the narrow slit produces wide diffraction of the light waves
to ensure that both S1 and S2 are illuminated

(ii)

slit S acts as a point source
S1 and S2 are illuminated from the same source, giving monochromatic light of the same wavelength
the paths from S to S1 and from S to S2 are of constant length, giving a constant phase difference between the waves

4(c)

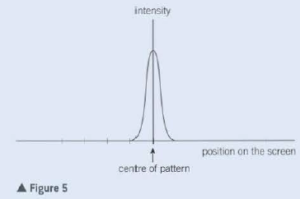
5 (a)

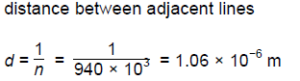
5(b)