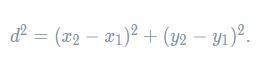This page contains the GCSE AQA Mathematics Percentage theorem Questions and their answers for revision and understanding Percentage theorem.
Pythagoras’ theorem is an equation that describes a relationship between the 3 sides of a right-angled triangle. The equation is
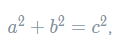
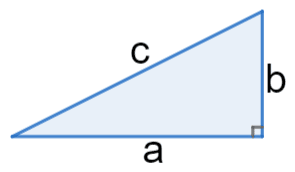
where cc is the hypotenuse (the longest side), and aa and bb are the two other sides – see below.
You will need to be able to use Pythagoras to find any missing side of a right-angled triangle. This may involve some algebraic rearranging but don’t worry, we’ll go through all of this in the examples below. On top of this, we will see how Pythagoras can be used to find the distance between two coordinates/points on a graph.
First of all, however, we need to prove Pythagoras’ theorem. There are literally more than 100 different ways to prove it, so here’s 1. To do this, let’s imagine we make 4 copies of the right-angled triangle above and arrange them in two different ways, both of which are shown below.
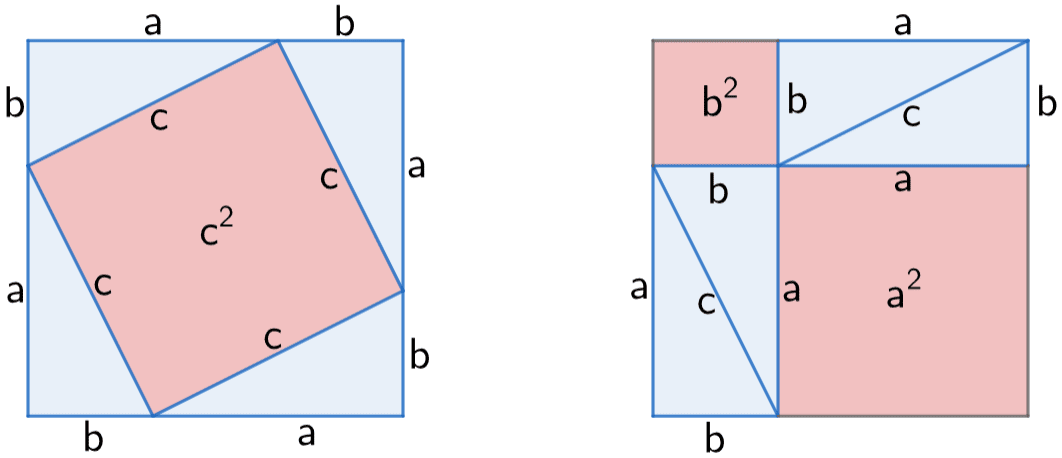
In the square on the left, you can see that they form a square with side-length a+b. Furthermore, the portion of the square that isn’t covered by the triangles (the red part) is a square with side-length cc, so must have area c2.
Now, look at the square on the right – the triangles are arranged differently, but they are still contained within one big square with side-length a+b. Furthermore, the portion of the square that isn’t covered by the triangles is broken up into two smaller squares: one with area a^2 and one with area b^2. Since both big squares are the same size and the triangles themselves haven’t changed, then the area not covered (red) must be equal in both squares. In other words, we have that
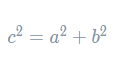Which is precisely the statement of Pythagoras’ theorem. Crushed it. Now we can get to using it.
Example: Below is a right-angled triangle. Find the length of the side marked x.
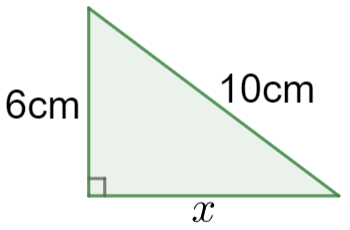
In order to find this using Pythagoras’ theorem, we need to work out which side corresponds to each of the letter a,b, and c in the equation. Actually, we really just need to recognise the longest side – 10cm – and make that cc, and then it doesn’t matter which one we make a or b. Let’s say a=x and b=6. Then, the equation becomes
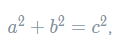
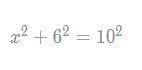
We know that 6^2=36 and 10^2=100, so we get
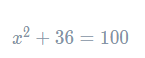
Now, subtracting 36 from both sides of the equation leaves us with
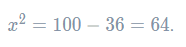
So, now that we have x^2=64, all that remains is to square root both sides:
So, the missing side-length is 8cm. This triangle is an example of a Pythagorean triple – a right-angled triangle where all 3 sides are whole number lengths. Many of these exist, but many right-angled triangles aren’t this nice. Often, the last step of taking a square root will result in an unpleasant decimal, or – if you are on the higher course – you may have to leave your answer as a surd.
The next example is how we use Pythagoras to find the distance between two coordinates.
Example: Work out the distance between (−4,−1) and (3,4). Give your answer to 1dp.
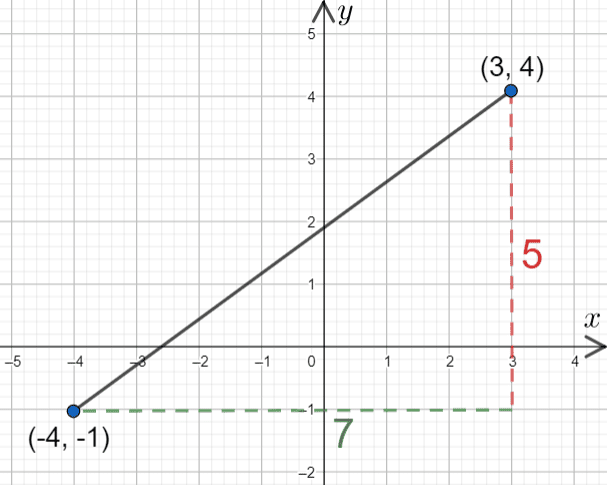
To do this, we will plot the two points on a pair of axes, draw a line between them, and then draw a triangle underneath – exactly the kind of triangle you would draw if you were finding the gradient (see: left). Then, also like if we were finding the gradient, work out how long each of the sides of this triangle is by counting the difference between the coordinates in both the x and y directions. It’s fine if this step is a very quick sketch, as long as your numbers are correct.
As you can see, because two the axes are at right-angles to each other, the triangle you draw will always be a right-angled triangle where we know the two shorter sides. So, in this case, the distance between the two points is cc, and let’s say a=5 and b=7. Then, the equation becomes
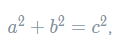
Now we have c^2=74, square rooting both sides, we get c=√74=8.6 to 1dp.
If you prefer formulas and don’t like graphs at all, then the distance, dd, between two coordinates (x1,y1) and (x2,y2) satisfies the equation
