This page contains the GCSE AQA Mathematics Circle Theorems Questions and their answers for revision and understanding Circle Theorems.
There are 8 circle theorems in total, and they’re all facts about angles/lengths in particular situations all involving circles. You should be familiar with them all to the point where a) you can see when they should be used, and b) you’re able to describe which one you’ve used with appropriate language. We’ll have a look at an example below but first, here’s all 8 of them.

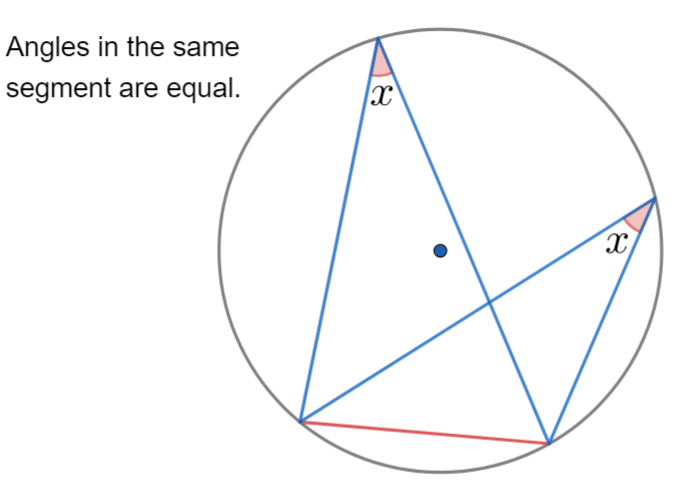

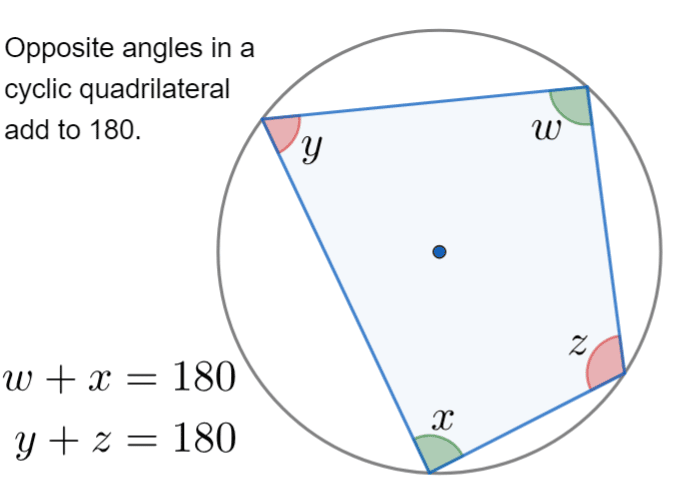
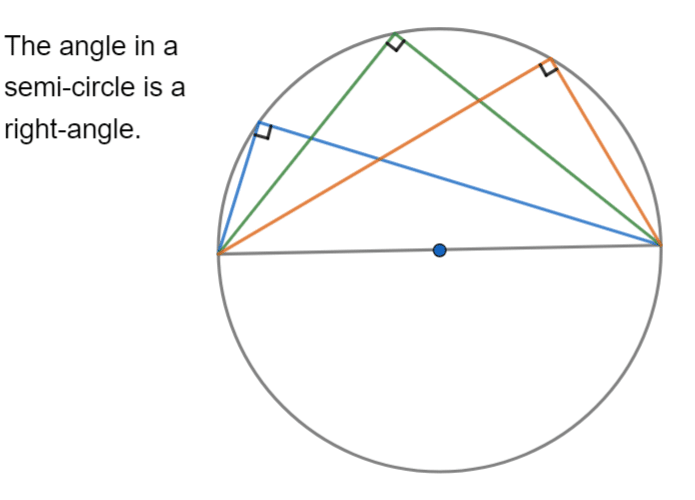
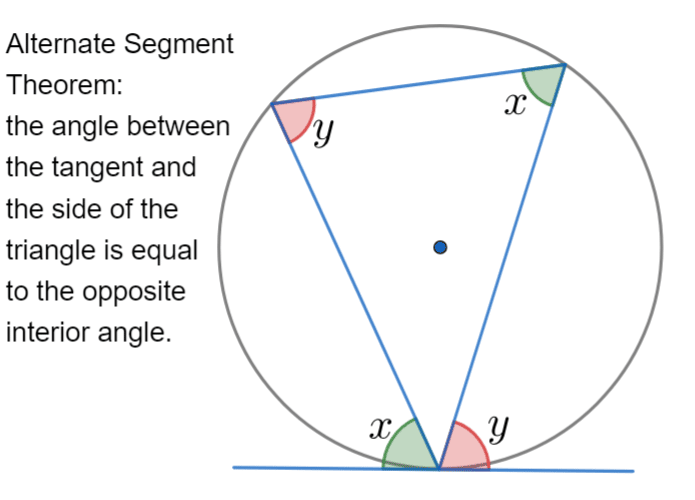
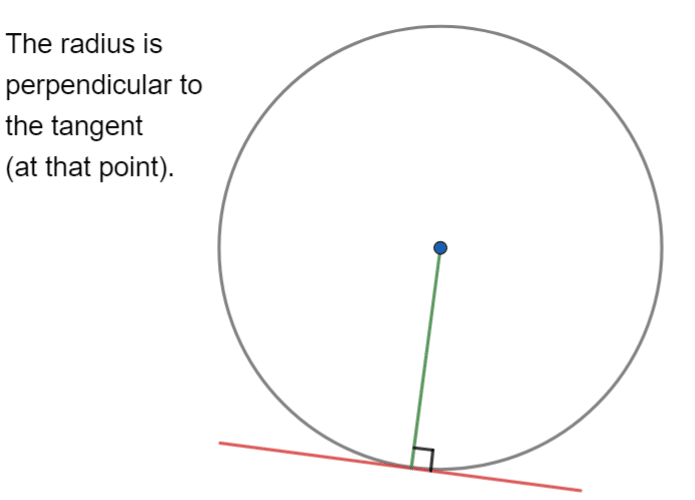

There is a small chance you might be asked how to prove these circle theorems, but it’s much more important that you understand how and when to use them so here we’ll be focussing on that.
Example: Below is a circle with centre C. BD is a diameter of the circle, A is a point on the circumference. What is the size of angle CBA?
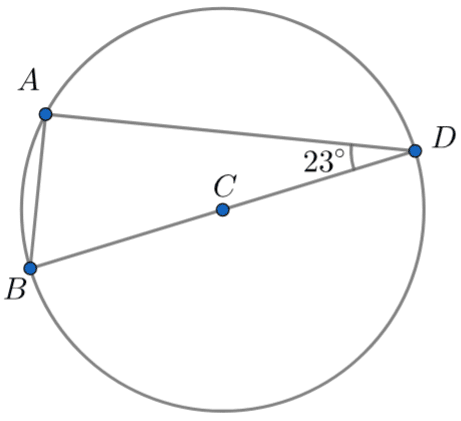
As we’re told that BD is a diameter of the circle, we know that triangle BAD is confined within the semi-circle. So, we can use the circle theorem that tells us the angle in a semi-circle is a right-angle to deduce that BAD =90°.
The question is asking for angle CBA, and now we know the other two angles in the triangle we can use the fact that angles in a triangle add to 180. Subtracting from 180, we get that
degree Angle CBA =180−23−90=67°
Example: Below is a circle with centre C. A, B, and D are points on the circumference. Angle BCD is 126° and angle CDA is 33°. Find angle ABC. You must show your workings.

When a question like this tells you to show our workings, you must state what circle theorem/geometry fact you use when you use it.
There’s no way for us to immediately find the angle we want, so we’re going to try to find the other angles in the quadrilateral ABCD. The first circle theorem we’re going to use here is: the angle at the centre is twice the angle at the circumference. The angle at the centre is 126, so degree angle BAD =126÷2=63°.
We now know two out of the four angles inside ABCD. To find a third, simply observe that angles around a point sum to 360, then we get that the angle at point C (the one inside ABCD) is 360−126=234°. Since the angles in a quadrilateral sum to 360, if we subtract the ones we know from 360 then find the angle we’re looking for.
Angle ABC =360−33−63−234=30°
