This page contains the GCSE AQA Mathematics algebraic fraction Questions and their answers for revision and understanding algebraic fraction.
An algebraic fraction is exactly what it sounds like: a fraction where you’ll find terms involving algebra on the numerator (top) and/or the denominator (bottom).
Whilst the introduction of algebra naturally makes fractions more complicated, it is important to remember that the rules which you learned regarding adding, subtracting, multiplying, dividing, simplifying, and generally manipulating normal fractions (i.e., the ones with only numbers in them) have not changed. First and foremost, it is still true that if you multiply (or divide) the top and bottom of a fraction by the same thing, then the value of the fraction does not change. Unless that thing is zero, in which case you’ll end up in heaps of trouble. What’s different now is that rather than just numbers, we might be multiplying by 5a5a or dividing by (x + 3)(x+3).
Simplifying
When simplifying fractions, the aim is to find a common factor in the top and the bottom and then cancel them out, e.g. we get that 6/8=3/4 by observing 6 and 8 have a common factor of 2, which we can then cancel from the top and bottom (in other words, we divide both numbers by it). Here, the aim is the same, but factors look a little different, and as the name suggests, we’re going to have to do some factorising to find them. Time to dust off all those factorising methods you learned.
Note: It may be the case that you have to do some expanding first before you can factorise. Bear this in mind when you’re having a go at the questions below.
Example: Simplify fully the fraction
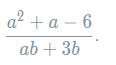
First, we will consider factorising the numerator. We see that is a quadratic, so observing that the numbers 3 and -2 multiply to make -6 and add to make 1, we get

Now, for the denominator:
This might seem insignificant, but what we now have is a factor of (a + 3)(a+3) in both the numerator and the denominator, which means we can cancel it. This looks like:
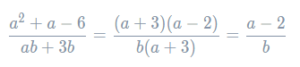
There is nothing else that the top and bottom have in common, so that must mean that we’re done.
Adding & Subtracting
When adding or subtracting algebraic fractions, the aim is still to find a common denominator. If you can, it might make your life a little easier to use the lowest common multiple of both the denominators in question as your common denominator. However, it isn’t always easy to know what this is so if you’re unsure, you can always safely use the product of both denominators instead (and to be honest, in algebraic fraction this often is the lowest common multiple anyway).
Example: Write as one fraction. Simplify your answer fully.
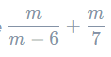
Our choice of common denominator will be the product of both denominators, which is
7×(m−6)=7(m−6)
We could expand the brackets, but it’s better to leave it factorised in case we can cancel anything later. So, our left-hand fraction is being multiplied by 7 on top and bottom, whilst our right-hand fraction is being multiplied by (m−6). So, we get
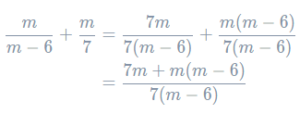
We have written it as one fraction, now we must simplify it fully. First, we should expand the brackets and collect terms on the top, which we can then factorise to see if there are any common factors we can cancel.
Denominator:
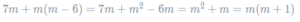
We cannot factorise this denominator further, so our fraction becomes
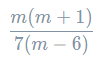
which shows no common factors in the top and bottom, so cannot be simplified further.
Multiplying & Dividing
Multiplying two fractions together remains a straightforward procedure even when algebra is involved: we simply multiply the numerators together and the denominators together (and then, as we have been doing, we simplify if possible). For division, the same rule of flipping the second fraction over and turning the division into a multiplication also remains.
Example: Simplify fully
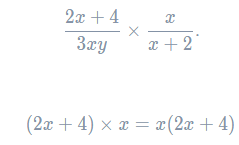
Multiply the numerators:
(2x+4)×x=x(2x+4)
The bonus here is that we don’t have to do any tricky factorisation since it’s already factorised.
Multiply the denominators:
3xy×(x+2)=3xy(x+2)
So our fraction is:
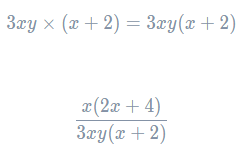
Firstly, we can cancel out the factor of xx on the top and bottom.
2x+4/3y(x+2)
Now, this might look like we have simplified it fully, but if we consider that 2x+4=2(x+2), then suddenly we have a common factor. We get:
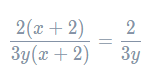
After cancelling the (x + 2) there are no more common factors, so we’re done.
